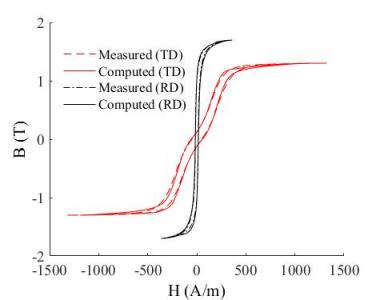
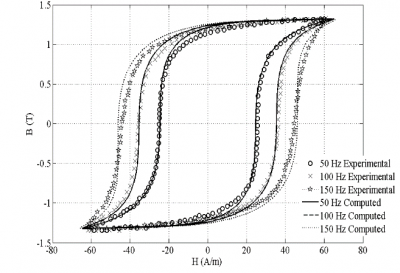
Grain-oriented (GO) materials are commonly used in magnetic circuits of power transformers. They provide a high permeable path for the main magnetic flux with minimum iron (or core) losses for magnetisation along their rolling direction (RD). These materials exhibit hysteretic, anisotropic, and dynamic features in their magnetisation process. Numerical simulations have to be carried out to optimise design, to pre-estimate the losses, and to test design performance under transient conditions. Hence, to model the magnetic part, a robust hysteresis model that is capable to consider thermo- magneto-mechanical loadings and amenable to numerical simulations is required. A number of hysteresis models are available in the literature. Among them, the Jiles-Atherton (JA) model and the Preisach model are widely used by researchers and engineers. The JA model is based on the energy dissipation during the magnetisation process that involves magnetic domain wall movement and rotation through pinning sites (defects) in the material. The basic JA model can model only magnetisation of isotropic materials and it is not robust to consider different operating conditions. The target of our research group is to propose a robust and comprehensive model that can model magnetic behaviour of these materials under different loading conditions. The basic JA model has been modified to incorporate the anisotropic behaviour (Fig. 1) based on equilibrium of domain patterns as described in Neel’s theory. The effect of frequency on hysteresis loops has also been analysed using a field separation approach (Fig. 2). Further, symmetrical minor loops along transverse direction (TD) are investigated in terms of major loop parameters using scaling power laws (Fig. 3). The dynamic part of the core loss contains eddy current loss and excess loss components. The excess loss exhibits anisotropic behaviour, which has been used to model hysteresis loops along any arbitrary direction. Hysteresis loops contain asymmetrical minor loops for harmonics in magnetisation. The number of minor loops in a hysteresis loop depends on the order of harmonics. Fig. 4 shows a hysteresis loop for magnetic flux density (B) with a 3 rd harmonic component. The parameters of the JA model have to be determined by using optimisation techniques like genetic algorithm, which is time-consuming and very complex in terms of computations. New algorithms are being developed to model highly nonlinear hysteresis curves using only few data points with less computational effort.